Student Survey
Evaluation of the Toolkit to Support Evidence-Based Algebra Instruction in Middle and High School
Appendix H. Student survey
OMB: 1850-0988
Appendix
H
Student Survey
Efficacy Study of the Toolkit to Support Evidence-Based Algebra Instruction
in Middle & High School
Student Survey
Spring 2025
Thank you for taking the time to complete this survey as part of a study about algebra instruction in [SCHOOL DISTRICT]. Through this survey, we want to learn about how you feel about your math abilities, your thoughts towards learning math, how math is taught in your Algebra 1 class, and how you approach solving algebra problems. In addition, we ask that you complete a few questions about solving math problems that are part of this survey. These questions will not affect your grade in math class and the results will not be shared with your teacher or other school staff.
The survey should take about 20 minutes to complete.
If you are unsure how to answer a question, please give the best answer you can rather than leaving it blank.
The information from this survey will be used for research purposes only and you won’t be identified in any way. All data collected for this study will be kept confidential, except as required by law. The researchers conducting this study follow the confidentiality and data protection requirements of the U.S. Department of Education’s Institute of Education Sciences (The Education Sciences Reform Act of 2002, Title I, Part E, Section 183). Your responses will not be shared with anyone outside of the study team.
This survey is voluntary. There are no risks or benefits for participating. You may skip any questions you do not wish to answer; however, we hope that you answer as many questions as you can.
This study has received Institutional Review Board (IRB) approval from Health Media Lab. An IRB reviews studies to make sure they are following ethical guidelines. Your school district has also approved of this study. If you have any questions about this study or your rights as a research volunteer, you can contact the study team at [EMAIL ADDRESS].
If you agree to participate in this survey, please check the box below and then start the survey.
I AGREE TO PARTICIPATE IN THIS SURVEY
Your beliefs about your math abilities
Please answer the following questions about your beliefs regarding your math abilities.
[ALL]
I.1. Please read each statement below and select the response which best describes your feelings for each statement. Mark one response for each row.
(Source: Mathematics Self-Efficacy and Anxiety Questionnaire (May, 2009), self-efficacy subscale, definition informed by Bandura (1978))
|
Strongly Disagree |
Disagree |
Not Certain |
Agree |
Strongly Agree |
I.1a. I feel confident enough to ask questions in my mathematics class. |
1 m |
2 m |
3 m |
4 m |
5 m |
I.1b. I believe I can understand the content in a mathematics course. |
1 m |
2 m |
3 m |
4 m |
5 m |
I.1c. I believe I can learn well in a mathematics course. |
1 m |
2 m |
3 m |
4 m |
5 m |
I.1d. I feel confident when taking a mathematics test. |
1 m |
2 m |
3 m |
4 m |
5 m |
I.1e. I believe I am the type of person who can do mathematics. |
1 m |
2 m |
3 m |
4 m |
5 m |
I.1f. I believe I can think like a mathematician. |
1 m |
2 m |
3 m |
4 m |
5 m |
[ALL]
I.2. Please read each statement below and select the response which best describes your feelings for each statement. Mark one response for each row.
(Source: Indiana Mathematics Belief Scale (Kloosterman and Stage, 1992) , Belief 1: Can solve time-consuming mathematics problems)
|
Strongly disagree |
Disagree |
Not certain |
Agree |
Strongly agree |
I.2a. Math problems that take a long time don’t bother me. |
1 m |
2 m |
3 m |
4 m |
5 m |
I.2b. I feel I can do math problems that take a long time to complete. |
1 m |
2 m |
3 m |
4 m |
5 m |
I.2c. I find I can do hard math problems if I just hang in there. |
1 m |
2 m |
3 m |
4 m |
5 m |
I.2d. If I can’t do a math problem in a few minutes, I probably can’t do it at all. |
1 m |
2 m |
3 m |
4 m |
5 m |
I.2e. If I can’t solve a math problem quickly, I quit trying |
1 m |
2 m |
3 m |
4 m |
5 m |
I.2f. I’m not very good at solving math problems that take a while to figure out. |
1 m |
2 m |
3 m |
4 m |
5 m |
Your beliefs about learning math
Please answer the following questions about your thoughts towards learning math.
[ALL]
II.1. Please read each statement below and select the response which best describes your feelings for each statement. Mark one response for each row
(Source: Indiana Mathematics Belief Scale (Kloosterman and Stage, 1992), Belief 3: Understanding concepts is important in mathematics)
|
Strongly Disagree |
Disagree |
Not Certain |
Agree |
Strongly Agree |
II.1a. Time used to investigate why a solution to a math problem works is time well spent |
1 m |
2 m |
3 m |
4 m |
5 m |
II.1b. A person who doesn't understand why an answer to a math problem is correct hasn't really solved the problem. |
1 m |
2 m |
3 m |
4 m |
5 m |
II.1c. In addition to getting a right answer in mathematics, it is important to understand why the answer is correct. |
1 m |
2 m |
3 m |
4 m |
|
II.1d. It's not important to understand why a mathematical procedure works as long as it gives a correct answer. |
1 m |
2 m |
3 m |
4 m |
5 m |
II.1e. Getting a right answer in math is more important than understanding why the answer works. |
1 m |
2 m |
3 m |
4 m |
5 m |
II.1f. It doesn't really matter if you understand a math problem if you can get the right answer. |
1 m |
2 m |
3 m |
4 m |
5 m |
[ALL]
II.2. Please read each statement below and indicate how true each statement is for you. Mark one for each row
(Source: Measuring MESH: Student surveys curated for the CORE districts (Transforming Education, 2016), growth mindset subscale)
|
Not at all true |
A little true |
Somewhat true |
Mostly true |
Completely true |
II.2a. My math ability is something that I can’t change very much. |
1 m |
2 m |
3 m |
4 m |
5 m |
II.2b. Challenging myself won’t make me any better at math. |
1 m |
2 m |
3 m |
4 m |
5 m |
II.2c. There are some parts of math I am not capable of learning. |
1 m |
2 m |
3 m |
4 m |
5 m |
II.2d. If I am not naturally good at math, I will never do well in it. |
1 m |
2 m |
3 m |
4 m |
5 m |
Algebra instruction and your algebra problem-solving approaches
This section focuses on the way algebra is taught in your classroom and the way(s) you approach solving algebra problems.
[ALL]
Please think about your Algebra 1 classroom over the last month and note how often your math teacher typically did any of the following activities. Please mark if your teacher never, rarely, sometimes, often, or very often did the following activities:
Mark one response for each row
(Source: all are new items informed by Star et al. (2015))
|
Never |
Rarely |
Sometimes |
Often |
Very often |
III.1. My math teacher shows me algebra problems that were already solved. |
0 m |
1 m |
2 m |
3 m |
4 m |
III.2. My math teacher asks me to talk about algebra problems that were already solved. |
0 m |
1 m |
2 m |
3 m |
4 m |
III.3. My math teacher asks me to find errors in algebra problems that have mistakes in them. |
0 m |
1 m |
2 m |
3 m |
4 m |
III.4. My math teacher asks me to compare at least two algebra problems that were already solved. |
0 m |
1 m |
2 m |
3 m |
4 m |
III.5. My math teacher asks me to pause, after first looking at an algebra problem, to give me time to think before solving the problem. |
0 m |
1 m |
2 m |
3 m |
4 m |
III.6. My teacher asks me to use correct math vocabulary when I talk about how an algebra problem might be solved. (For example, vocabulary could include factor, use the distributive property, and subtract 5 from both sides of the equation). |
0 m |
1 m |
2 m |
3 m |
4 m |
III.7. My teacher asks me to describe the representations used in different types of algebra problems. (For example, representations could include word problems, equations, graphs, or diagrams). |
0 m |
1 m |
2 m |
3 m |
4 m |
III.8. My teacher asks me to think about more than one strategy for an algebra problem before I start solving the problem. |
0 m |
1 m |
2 m |
3 m |
4 m |
III.9. My teacher asks me to explain why the way I used to solve an algebra problem works. |
0 m |
1 m |
2 m |
3 m |
4 m |
III.10. My math teacher asks me to compare at least two different ways to solve an algebra problem. |
0 m |
1 m |
2 m |
3 m |
4 m |
[ALL]
For the following statements, please think about how often you typically used each of the algebra problem-solving strategies over the last month. Please mark if you never, rarely, sometimes, often, or very often used each algebra problem-solving strategy.
Mark one only for each row
(Source: all are new items informed by Star et al.(2015))
|
Never |
Rarely |
Sometimes |
Often |
Very often |
III.11. When I am solving a new algebra problem, I look at other, similar algebra problems that have already been solved. (for example, when solving a one-step equation, I look at other problems that have been solved with one step). |
0 m |
1 m |
2 m |
3 m |
4 m |
III.12. When I am solving a new algebra problem, I look at other, similar algebra problems that have been solved with mistakes. |
0 m |
1 m |
2 m |
3 m |
4 m |
III.13. I ask myself questions to help understand an algebra problem. (For example, “what am I being asked to do in this problem”; or “what am I trying to solve for?”) |
0 m |
1 m |
2 m |
3 m |
4 m |
III.14. I know how to describe how to solve an algebra problem using correct math vocabulary. |
0 m |
1 m |
2 m |
3 m |
4 m |
III.15. When algebra problems include multiple representations (i.e., word problems, graphs, diagrams, equations, etc), I understand how these multiple representations connect to each other. (For example, I can connect the slope on the graph of a line to the slope in the equation for the same line). |
0 m |
1 m |
2 m |
3 m |
4 m |
III.16. I try to think of more than one way to solve algebra problems. |
0 m |
1 m |
2 m |
3 m |
4 m |
III.17. I solve the same type of algebra problem in more than one way. |
0 m |
1 m |
2 m |
3 m |
4 m |
III.18. I explain why I selected a particular method, instead of a different method, to solve a problem. |
0 m |
1 m |
2 m |
3 m |
4 m |
Algebra problems to solve
Please read through the following algebra problems and answer the questions for each one. Your responses to these algebra problems will not affect your grades in your Algebra 1 class.
For
problems IV.1 – IV.4, consider the equation

[ALL]
Edna
solved this equation,
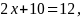 using the following steps: (Source:
new item informed by Star
et al. (2015) and Durkin
et al. (2023))
using the following steps: (Source:
new item informed by Star
et al. (2015) and Durkin
et al. (2023))

 I factored out a 2 from the left side of the equation.
I factored out a 2 from the left side of the equation.
 I divided both sides by 2.
I divided both sides by 2.
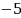
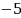 I subtracted 5 from both sides.
I subtracted 5 from both sides.
 Here is my answer.
Here is my answer.
IV.1. Edna substitutes her solution into the original equation to check her work. Which of the following statements is correct, as Edna checks her work?
Mark one only (Source: new item informed by Star et al. (2015) and Durkin et al. (2023)
Edna’s solution is correct, because
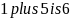 .
.Edna’s solution is incorrect, because
 times
times
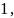 plus
plus
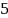 is
is
 ,
not
,
not
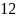 .
.Edna’s solution is correct, because
 times
times
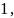 plus
plus
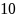 is
is
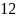 .
.Edna’s solution is incorrect, because
 times
times
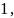 plus
plus
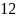 is
is
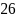 ,
not
,
not
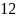 .
.None of the above.
[ALL]
IV.2. Which of the following statements is true about Edna’s method?
Mark one only (Source: new item informed by Star et al. (2015) and Durkin et al. (2023)
Edna’s method is not mathematically valid because she should have subtracted
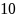 from both sides as a first step.
from both sides as a first step.Edna’s method is not mathematically valid because she should have divided both sides by
 as a first step.
as a first step.Edna’s method is mathematically valid for this equation but would not be helpful for solving the equation
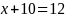 .
.Edna’s method is mathematically valid and would be helpful for solving any other linear equation.
[ALL]
IV.3.
Frank
is trying to solve the equation
 and he wants to use Edna’s method. Which of the following
should be his first step?
and he wants to use Edna’s method. Which of the following
should be his first step?
Mark one only (Source: new item informed by Star et al. (2015) and Durkin et al. (2023)
He should add
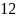 to both sides.
to both sides.He should divide both sides by
 .
.He should rewrite
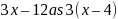 .
.He should rewrite
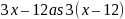 .
.None of the above.
[ALL]
Gail
solved the equation
 with the following steps:
(Source:
new item informed by Star
et al. (2015) and Durkin
et al. (2023))
with the following steps:
(Source:
new item informed by Star
et al. (2015) and Durkin
et al. (2023))


 I
subtracted 10 from both sides.
I
subtracted 10 from both sides.


 I
divided both sides by 2.
I
divided both sides by 2.
 Here
is my answer.
Here
is my answer.
IV.4. Which of the following statements is true about Gail’s method?
Mark one only (Source: new item informed by Star et al. (2015) and Durkin et al. (2023)
Gail’s method and Edna’s method are both mathematically valid, but Gail’s method is always better when solving any linear equation.
Gail’s method is mathematically valid, and Edna’s method is not mathematically valid.
Edna’s method is mathematically valid, and Gail’s method is not mathematically valid.
Gail’s method and Edna’s method are both mathematically valid and both are helpful ways to solve linear equations.
None of the above.
Use the correct and incorrect responses in the box below to answer IV.5.
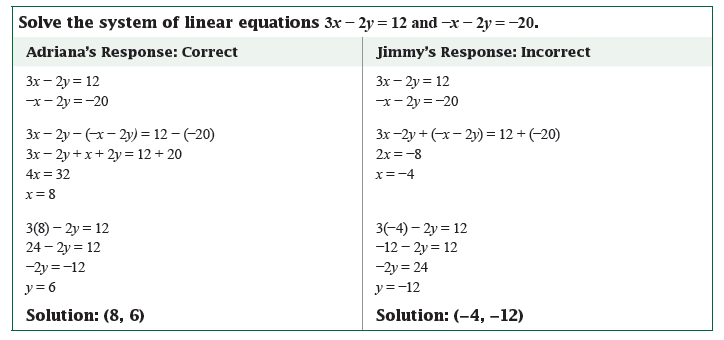
[ALL]
IV.5. What was the initial mistake that led to Jimmy’s incorrect answer?
Mark one only (Source: new item informed by Star et al. (2015) and Durkin et al. (2023)
In Jimmy’s second step (line
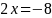 ),
he made a calculation error when combining like terms.
),
he made a calculation error when combining like terms.In Jimmy’s final step (line
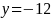 ),
he divided by
),
he divided by
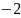 when he should have divided by
when he should have divided by
 .
.In Jimmy’s first step, (line
 ),
he added the two equations together when he should have subtracted
the second equation from the first.
),
he added the two equations together when he should have subtracted
the second equation from the first.In Jimmy’s fourth step (line
 ),
he substituted incorrectly.
),
he substituted incorrectly.
[ALL]
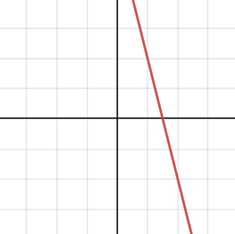
IV.6. Representations of four different linear functions are shown below. Which linear function has a slope that is not equal to the slope of the other three functions? Mark one only (Source: new item informed by Star et al. (2015)

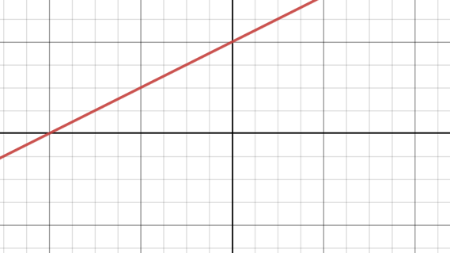
-

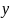


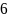

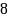

…
…

[ALL]
IV.7. Which of the below linear functions has an x-intercept that is equal to its y-intercept, where a and b are both positive numbers?
Mark one only (Source: new item informed by Star et al. (2015)
The line that goes through the points
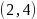 and
and

The line that goes through the points
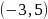 and
and

The line that goes through the points
 and
and

The line that goes through the points
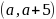 and
and

[ALL]
IV.8. Which of the following linear functions has a positive slope?
Mark one only (Source: new item informed by Star et al. (2015)

The line through the points
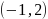 and
and
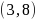
The line with x-intercept of
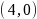 and y-intercept of
and y-intercept of
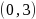
[ALL]
IV.9. Three of the following equations have very similar mathematical structure, while the fourth equation has a different mathematical structure. Select the equation that has a different structure than the other three.
Mark one only (Source: new item informed by Star et al. (2015)
[ALL]
IV.10. For the following problems, two mathematical statements are given for each problem. For each pair of statements, select whether (1) Statement 1 uses more precise and valid mathematical language, (2) Statement 2 uses more precise and valid mathematical language, or (3) both statements’ use of mathematical language is the same in terms of precision and validity.
Mark one for each row (Source: new items informed by Star et al. (2015))
|
Which statement uses more precise and valid mathematical language? |
||
|
Statement 1 |
Statement 2 |
Both statements use equally precise and valid mathematical language |
IV.10a.
Statement
1:
Solve
the expression
Statement
2:
Evaluate
the expression
|
1 m |
2 m |
3 m |
IV.10b. Statement
1:
One
step for solving the equation
Statement
2:
One
step for solving the equation
|
1 m |
2 m |
3 m |
IV.10c.
Statement
1:
To
figure out what
Statement
2:
To
figure out what
|
1 m |
2 m |
3 m |
IV.10d.
Statement
1:
Solving
the equation
Statement
2:
Solving
the equation
|
1 m |
2 m |
3 m |
For problems IV.11 – IV.14, consider the following system of linear equations problem and Zach and Yolanda’s solutions to this problem.
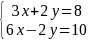
Zach and Yolanda solved the above system of linear equations problem.
The first steps of their solutions are shown below.
Zach’s solution: Yolanda’s solution:



[ALL]
IV.11. Which of the following statements is true about Zach’s method?
Mark one only (Source: new item informed by Star et al. (2015)
Zach is using the “substitution” method for solving systems of linear equations.
Zach is using the “elimination” method for solving systems of linear equations.
Zach has made a mistake in his method.
None of the above.
[ALL]
IV.12. Which of the following is true about Yolanda’s method?
Mark one only (Source: new item informed by Star et al. (2015)
Yolanda is using the “substitution” method for solving systems of linear equations.
Yolanda is using the “elimination” method for solving systems of linear equations.
Yolanda has made a mistake in her method.
None of the above.
[ALL]
IV.13. Which of the following statements is true?
Mark one only (Source: new item informed by Star et al. (2015)
Zach’s method is a better method for solving this particular system of equations because it takes fewer steps.
Yolanda’s method is a better method for solving this particular system of equations because it takes fewer steps.
Both Zach’s and Yolanda’s methods are equally valid and efficient ways to solve this particular system of equations; neither method is better than the other.
None of the above.
[ALL]
IV.14. If you were asked to solve the new system of linear equations problem shown below, which of the following statements is true?
Mark one only (Source: new item informed by Star et al. (2015)
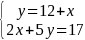
Elimination method is a better, more efficient method for solving this second system of equations.
Substitution method is a better, more efficient method for solving this second system of equations.
Both elimination and substitution methods are valid and efficient ways to solve this second system of equations; neither method is better than the other.
None of the above.
Thank you for completing the student survey!
| File Type | application/vnd.openxmlformats-officedocument.wordprocessingml.document |
| Author | Caroline Lauver |
| File Modified | 0000-00-00 |
| File Created | 2024-07-26 |
© 2025 OMB.report | Privacy Policy

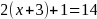

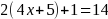
 when
when
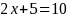 is to add
is to add
 is equal to, you flip the
is equal to, you flip the
 and then multiply
and then multiply
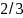 times
times
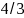 .
.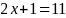 means finding the value of
means finding the value of